x³y³=(X+Y)(X²-XY+Y²) xy=1 x³y³=X²-XY+Y² x3y33xy=X²2XY+Y²=(xy)²=1Near (x, y)=(3245, 197), (309, 845), (25, 9) and (2365, 15) And since the two equations can be combined into a quartic in x, (or a quartic in y) and a quartic has 4 roots in the complex numbers and we have found 4 real roots then these are the only solutions even if you allowed x and y to be complex numbers (x y z) 2 = x 2 y 2 z 2 2xy 2yz 2zx (x y) 3 = x 3 y 3 3xy(x y) (x – y) 3 = x 3 – y 3 – 3xy(x – y) x 3 y 3 z 3 – 3xyz = (x y z)(x 2 y 2 z 2 – xy – yz – zx;
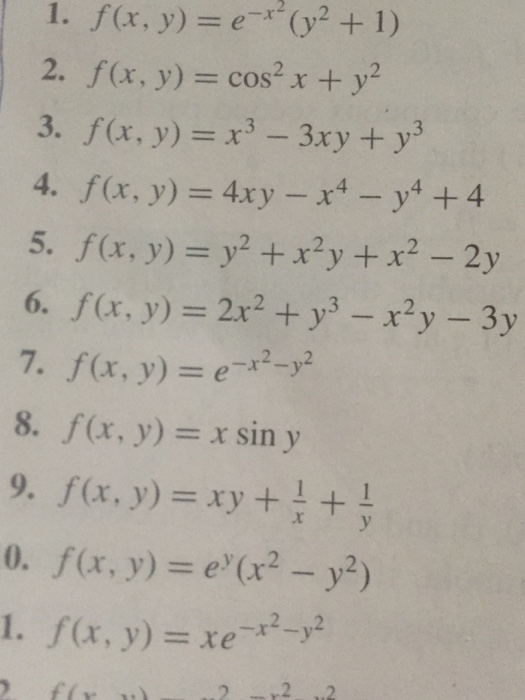
F X Y E X 2 Y 2 1 F X Y Cos 2 X Y 2 Chegg Com
(x+y)^3=x^3+y^3+3xy(x+y)
(x+y)^3=x^3+y^3+3xy(x+y)-1 How do you simplify the cube of a binomial?Telangana SCERT Class 9 Math Chapter 2 Polynomials and Factorisation Exercise 25 Math Problems and Solution Here in this Post Telanagana SCERT Class 9 Math Solution Chapter 2 Polynomials and Factorisation Exercise 25
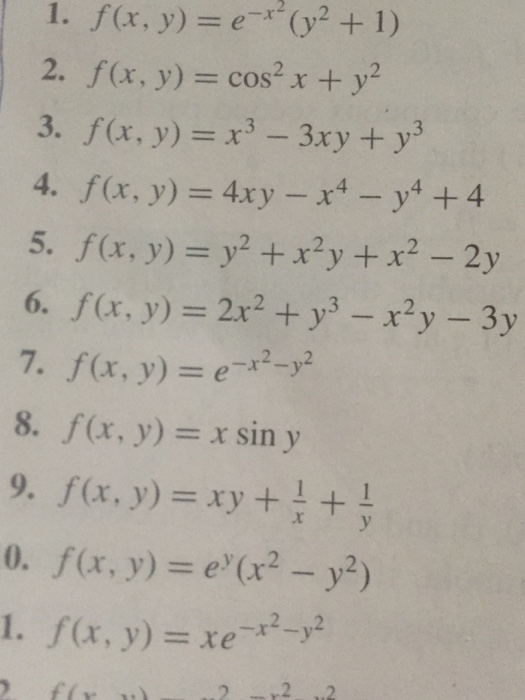



F X Y E X 2 Y 2 1 F X Y Cos 2 X Y 2 Chegg Com
If x y = 12, and xy = 27, then find the value of x3 y3 Welcome to Sarthaks eConnect A unique platform where students can interact with teachers/experts/students to get solutions to their queries If xy = 4 and xy = 21 then find the value of x3 y3 Maths Polynomials NCERT Solutions;(x y) 3 = x 3 y 3 3xy(x y) (x – y) 3 = x 3 – y 3 – 3xy(x – y) x 3 y 3 z 3 – 3xyz = (x y z) (x 2 y 2 z 2 – xy – yz – zx) Share these Notes with your friends Prev Next > You can check our 5step learning process Classes Class 6 Class 7 Class 8 Class 9 Class 10 Class 11 Class 12 NEET Subjects Physics
(i) x 3 y 3 = (x y)(x 2 – xy y 2) (ii) x 3 – y 3 = (x – y) (x 2 xy y 2) Solution (i) We know that, (x y) 3 = x 3 y 3 3xy (x y) ⇒ x3 y3 = (x y)3 – 3xy (x y) = (x y) (x y) 2 – 3xy = (x y) x 2 y 2 2xy – 3xy = (x y) x 2 y 2 – xy = RHS Hence proved, (ii) We know that, (x – y) 3 = x 3 – y 3 – 3xy (x – y) x 3 – y 3Philip Macdonald Answered 1 year ago Author has 234 answers and 413K answer views xy =7, xy =1 (xy)^3 = x^3 3x^2*y 3x*y^2 y^3 = x^3y^3 3xy (xy) Then 7^3 = x^3y^3 3*1*7, ie, x^3y^3 = 7^3 7*3 = 7 (7^2 3) = 7*46 =322 143 views Sponsored by Jumbo Privacy & Security = (4a – 3b) 3 ∵ (x – y) 3 = x 3 – y 3 – 3xy(x – y) = (40 – 3b) (4a – 3b) (4a – 3b) Question 3 What are the possible expressions for the dimensions of a cuboid whose volume is given below ?
0 Follow 0 A K Daya Sir, added an answer, on 25/9/13 A K Daya Sir answered this x 3 y 3 = (x y) (x 2 xy y 2 ) this formula can be derived from (x y) 3 = x 3 y 3 3xy (x y) x 3 y 3 = (x y) 3 3xy (x y) x 3 y 3 = (x y) (x y) 2 3xy = (x y) x 2 y 2 2xy 3xy = (x y) (x 2 xy y 2 ) Was this answer helpful?X 0 =1 x a y a = (xy) a, 2 2 3 2 = 6 2;Kuchaman Maths Guru !



Factorize X X 3 Y 3 3xy X Y Sarthaks Econnect Largest Online Education Community




Let F X Y X 3 3x 2y 3 2y 2 We Find Fx X Y 3x 2 Chegg Com
= x^3 3x^2y 3xy^2 y^3 = x^3 y^3 3xy(x y) Also, Read Cube of a Binomial Cube of Sum of Two Binomials Examples 1 Determine the expansion of (x 2y)^3 Solution The given expression is (x 2y)^3 We have an equation on cubes like (x y)^3 = x^3 y^3 3xy(x y) By comparing the above expression with the (x y)^3 Here, x2y=2 y=1 Hence, putting the value of y again in any of the above three equations will give the value of x So, taking equation x=y5 x=1–5 x=4 So the final answers are x=4 and y=1 For any clarification, please update in comments And if you liked the answer do upvote and sorry for any grammatical mistakes35 (1) Upvote (1) Choose An Option That Best Describes Your Problem Answer not in Detail Incomplete Answer Answer Incorrect Others Answer not in Detail Incomplete Answer Answer Incorrect
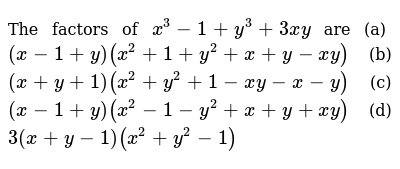



The Factors Of X 3 1 Y 3 3x Y Are A X 1 Y X 2 1 Y 2 X Y
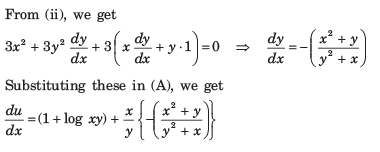



If U X Log Xy Wherex3 Y3 3xy 1 Then Du Dx Is Equal Toa B C D Correct Answer Is Option A Can You Explain This Answer Edurev Electronics And Communication Engineering Ece
Ĺet x^yy^x=(xy)^3=x^3y^33xy(xy) Or,x^yy^x=x^3y^39xy again x=3y so x^3y^39xy=(3y)^3y^39(3y)y=2727y9y^2y^3y^39y^227=5427y Now we got 54 27y=27, or 27y=27,y=1 Then x=31=2 The values of x& y only satisfy xy=3 and doen't satisfy x^yy^x=27 So there is no solution for the values of x & y Answer (xy)^3 = (xy)^2 (xy) = (x^2y^22xy) (xy) = x^3xy^22x^2yx^2yy^32xy^2 = x^3y^33x^2Without doing the maths the answer is very clearly yes!
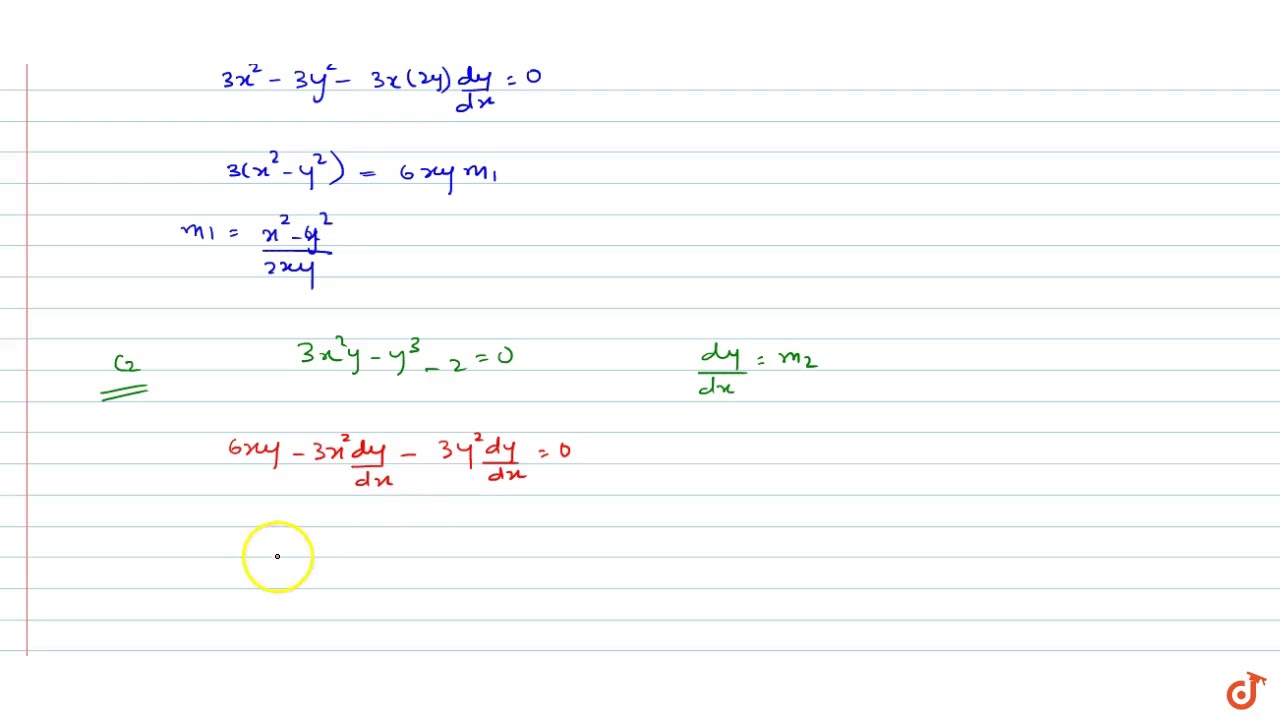



The Two Curves X 3 3xy 2 2 0 And 3x 2y Y 3 2 0 Youtube
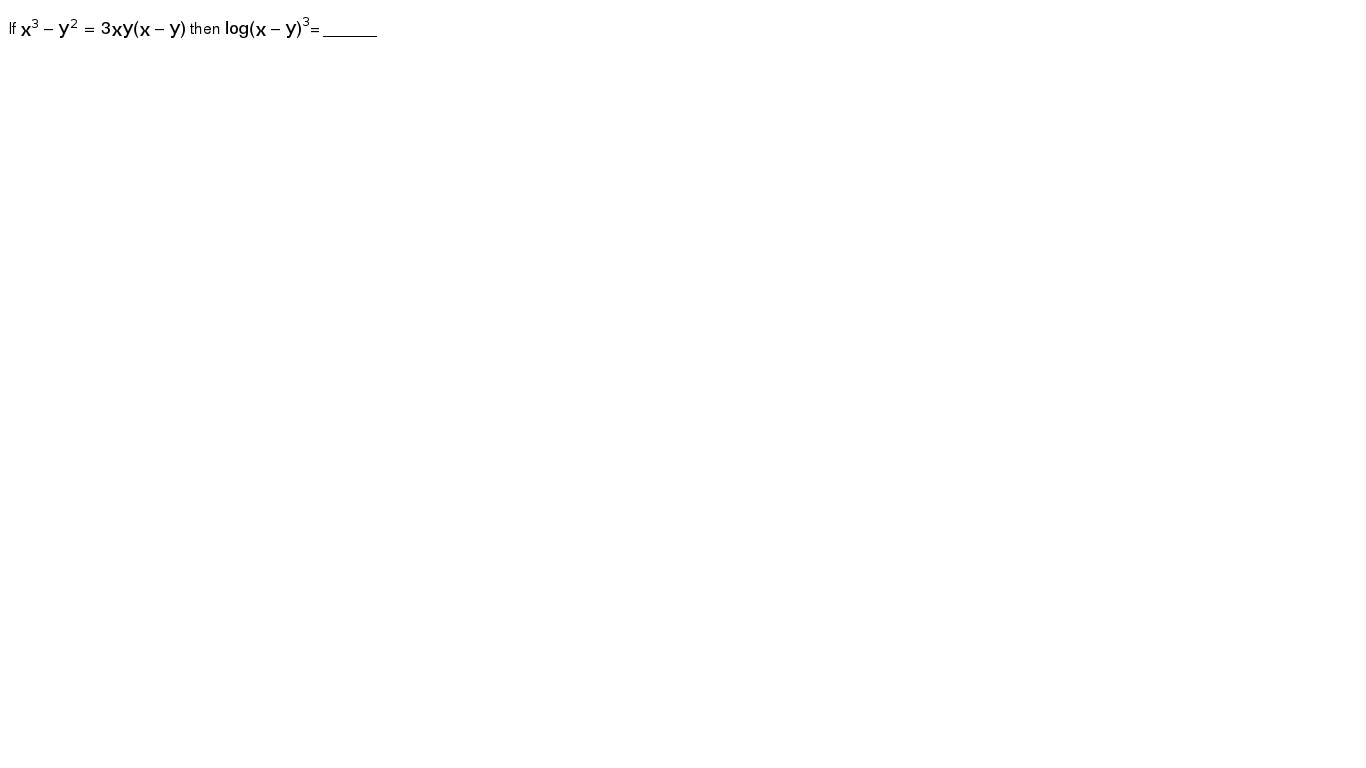



If X 3 Y 2 3xy X Y Then Log X Y 3
Start your 48hour free trial to unlock this answer and thousands more Enjoy eNotes adfree and cancel anytimeTutor Contact tutor 7 months ago Use identity ( a b)^3 = a^3 b^3 3ab (a b ) Put a= x and b= y ( x y)^3 = x^3 y^3 3xy ( x y ) In further step 3xy can be multiplied inside the bracket The answer is 👍 HelpfulVolume = 12ky 2 8ky – k Solution We have, volume = 12ky 2 8ky – k = 4k(3y 2 2y – 5) = 4k(3y 2 5y – 3y – 5)




X 9y 3xy X Y Answer It Plz With Full Maths Brainly In




If X Y 1 Then Find The Value Of X3 Y3 3xy Brainly In
In mathematics, the cube of sum of two terms is expressed as the cube of binomial x y It is read as x plus y whole cube It is mainly used in mathematics as a formula for expanding cube of sum of any two terms in their terms ( x y) 3 = x 3 y 3 3 x 2 y 3 x y 2Ĺet x^yy^x= (xy)^3=x^3y^33xy (xy) Or,x^yy^x=x^3y^39xy again x=3y so x^3y^39xy= (3y)^3y^39 (3y)y=2727y9y^2y^3y^39y^227=5427y Now we got 54 27y=27, or 27y=27,y=1 Then x=31=2 The values of x& y only satisfy xy=3 and doen't satisfy x^yy^x=27 So there is no solution for the values of x & yThere are two variables and one equation so there will be an infinite number of solutions Let's solve for y xy = xy x = xy y factor out the y x = y (x1) x x − 1 = y so y = x x − 1 x ≠ 1



F X Y E X 2 Y 2 1 F X Y Cos 2 X Y 2 Chegg Com
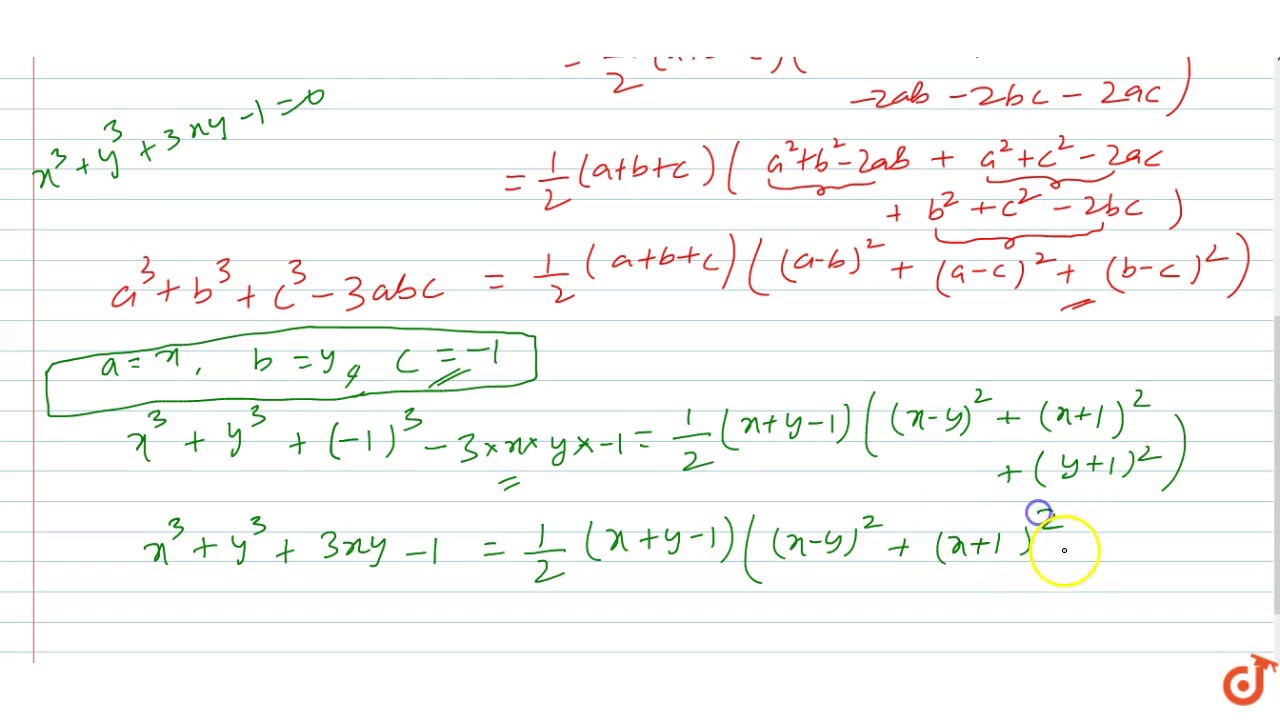



Q 16 Locus All Point P X Y Satisfying X 3 Y 3 3xy 1 Consists Of Union Of A A Youtube



0 件のコメント:
コメントを投稿